|
Problem 21.1
The process of gene conversion “converts” one allele in a heterozygote to another (p. 588). If conversion is equally likely in either direction (i.e., if Aa produces an a or an A gamete by conversion with equal probability), then there is no net effect on the population. However, the process is usually biased and so causes a change in allele frequencies that is similar to selection. NOTE 21A
Suppose that gene conversion occurs in APAQ heterozygotes at a rate c = 0.001, and always converts the AQ allele to AP.
|
| *i) |
If AP is initially at p = 0.01, what will be the change in allele frequency in one generation? HINT 21A
|
| **ii) |
*C28* How long will it take for AP to reach a frequency of 99%? HINT 21B
|
|
Problem 21.2
A hermaphroditic flowering plant is polymorphic for alleles that cause male sterility; these alleles are carried on the mitochondrial genome. Initially, 1% of plants are male-sterile. Male-sterile plants cannot pollinate other plants, but they can divert resources from pollen production and so produce 50% more seeds. Under uncrowded conditions, male-fertile plants produce on average ten surviving seeds per generation, whereas male-sterile plants produce 15.
|
| *i) |
How quickly will male sterility alleles increase? What will happen to the population as male sterility becomes common? HINT 21C
|
| **ii) |
When pollen becomes scarce, the chance that an ovule will be fertilized decreases. This chance is 1 – exp(–10q), where 0 < q < 1 is the amount of pollen produced by the population relative to that produced by a fully fertile population (Fig. P21.1). How frequent must male-sterile plants become for the population to become extinct? How long will this take? HINT 21D
|
| **iii) |
If a fraction p of plants are male-sterile, and if the population is maintaining a stable size, how many offspring will a male-fertile plant produce by pollinating others? How many will it produce through its ovules? HINT 21E
|
| **iv) |
After male sterility has reached p = 99%, a dominant allele that restores male fertility arises on an autosome. How quickly will such an allele increase?
|
| **v) |
What will be the final outcome? HINT 21F
|
|
Problem 21.3
In the early 1980s, Drosophila simulans in Southern California became infected with Wolbachia. These bacteria are transmitted from the mother. Their only obvious effect is that when uninfected females mate with infected males, those females become sterile and leave no offspring.
|
| *i) |
If p = 10% of flies are infected, what fraction will be infected in the next generation? HINT 21G
|
| **ii) |
How will the frequency increase over the following few generations? How will it increase if initially 1% of flies are infected? HINT 21H
|
| ***iii) |
Suppose that infected females produce s = 5% fewer surviving offspring. Will Wolbachia be able to spread? HINT 21I
|
|
Problem 21.4
A virus carries two genes: One codes for its protein coat, and one codes for its polymerase. Both genes must be present in an infected cell if the virus is to be able to reproduce, but individual viruses that have lost one of the genes can be replicated more quickly. Three types are found in the population: intact virus with both genes (CP) and virus that have only one of the genes (C, P).
A very simple model of this system tracks the average numbers of each type that enter infected cells (nC, nP, nCP). Their total number is N = nC + nP + nCP. The number of viruses that emerge and infect cells at the beginning of the next generation is
N* = (nC + nCP)(nP + nCP)12 exp(–N).
This is proportional to the number of coat genes present (nC + nCP) and to the number of polymerase genes present (nP + nCP): If either is zero, no virus can be produced. It is also proportional to a decreasing function of total numbers, 12 exp(–N), which represents density-dependent regulation of population size. (Numbers are measured on an arbitrary scale, and random fluctuations are ignored.) Within cells, nC and nP produce α times as many viruses as the intact genotype, nCP (α > 1). Thus, the proportion of type C that emerge is
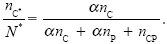
NOTE 21B
|
| **i) |
What is the equilibrium of nCP when only intact virus is present? HINT 21J
|
| *ii) |
Will types C or P be able to invade such a population?
|
| *iii) |
What will the equilibrium number be if only C and P are present? HINT 21K
|
| **iv) |
Will type CP be able to invade a population that has only C and P present?
|
|
Problem 21.5
Male mice that are heterozygous for the t allele (denoted t/+) produce almost entirely t sperm; male mice homozygous for t are sterile, but assume for the moment that t/t females are fertile.
|
| *i) |
How rapidly will the t allele increase from low frequency? HINT 21L
|
| **ii) |
Once most males are tt, will the t allele keep increasing toward fixation and, eventually, extinction of the population?
|
| **iii) |
Now, suppose that the t allele is tightly linked to a recessive lethal  , producing a new allele t. Mice of both sexes homozygous for this allele die early; they can be replaced by viable genotypes, and so the mother does not lose fitness. If this new t allele enters a wild-type population of +/+ mice, how fast will it increase? What will be the final equilibrium?
|
| **iv) |
What would happen if a t allele were introduced into a population of t/+ mice? Can the t allele displace the t allele. HINT 21M
|
|
Problem 21.6
Haldane remarked that he would lay down his life for two brothers or eight cousins. This problem explores the genetic basis for this statement.
|
| **i) |
Imagine an autosomal allele that increases its bearer’s mortality by 10% but reduces a brother or sister’s mortality by 30%. Would such an allele spread? HINT 21N
|
| ***ii) |
Now, suppose that the allele is carried on the X chromosome in a mammal. Would it still spread? HINT 21O
|
| **iii) |
Would it spread if it only reduced mortality of sisters? HINT 21P
|
|
Problem 21.7
This problem shows how we can predict the sex ratio in species of fig wasp (see Fig. 21.22). NOTE 21C
|
| **i) |
The main force influencing the sex ratio is the advantage to the rarer sex, which tends to produce an even sex ratio. If there is a proportion q of females, and p = 1 – q of males, in the population, then what is the fitness of a male relative to that of a female?
|
| **ii) |
Now, suppose that the population is inbred, such that the probability of identity by descent between a gene in the mother and a gene in the sperm, which she carries, is F. How many copies of her genes will a female pass on if she allows an egg to be fertilized, producing a daughter? How many will she pass on if she leaves the egg unfertilized, producing a son? HINT 21Q
|
| *iii) |
What is the equilibrium sex ratio? HINT 21R
|
| **iv) |
Now, suppose that there are only n foundress females within a fig. Thus, there is local mate competition between the males to fertilize the available eggs. Now, a daughter will carry out of the fig a gene from a male, who may be her brother. Taking this into account, how many genes does a female expect to pass on in total if she produces a daughter? HINT 21S
|
| **v) |
If a female produces a son, there is a chance that this son will displace his brother and give no net gain. Taking this into account, how many genes does a female expect to pass on in total if she produces a son? HINT 21T
|
| **vi) |
Now, what is the equilibrium sex ratio? Sketch graphs that show how sex ratio varies with the degree of inbreeding, F, and the number of foundresses, n. HINT 21U
|
|
Problem 21.8
A plant produces seed pods that each contains ten fertilized ovules. The future prospects of each embryo depend on how much resource it is allocated. The plant has 5 units of resource available for each pod; z1 + z2 + ··· + z10 = 5 is allocated to each embryo. Half the embryos have high viability and have expected relative fitness 5z/(1 + z); the other half have low viability and have one-fifth the expected relative fitness, z/(1 + z). Thus, there are diminishing returns to allocating more resources: Even if z is very large, fitness cannot exceed 5 for high-viability seeds or 1 for low-viability seeds. So, other things being equal, an even allocation across each class of seed is best (Fig. P21.2). NOTE 21D
|
| *i) |
What is the total fitness of the offspring from a pod if resources are allocated evenly?
|
| *ii) |
What is the total fitness if resources are allocated evenly over the five high-quality seeds and the low-quality seeds are aborted?
|
| **iii) |
Would the fitness be greater if some resource were distributed evenly over the low-quality seeds? HINT 21V
|
| **iv) |
Assume that each ovule is pollinated by a different male parent. What allocation of resources would maximize the fitness of a gene from the father? HINT 21W
|
| *v) |
Now, consider an outbred gene that was inherited from the mother and is expressed in a high-quality embryo. How many copies will it pass on if all resources are allocated to that embryo?
|
| **vi) |
How many copies will be passed on if some resources are allocated to that embryo and some to the four fitter siblings? HINT 21X
|
| **vii) |
Is this the optimal allocation of resources from the point of view of a gene inherited from the mother? HINT 21Y
|
| **viii) |
Finally, consider an allele inherited from the mother in a low-quality embryo. What would be the net fitness of this allele if all resources were allocated to its embryo? In that case, would there be a gain by allocating some resource to the five high-quality siblings? If all resources were allocated to those five, what would be the net fitness? What is the optimal strategy? HINT 21Z
|
|
Problem 21.9
A population is divided into large numbers of demes, each of N = 1000 haploid individuals. These exchange migrants very rarely (one individual every 100 generations, or Nm ~ 0.01, say), but occasionally become extinct. Empty patches are then immediately recolonized by a single individual from some other deme. There are two alleles, AP and AQ, at a gene, A, which have the same relative fitness when they compete within demes. However, demes that are fixed for AP become extinct at a rate of λP = 5% per generation, whereas demes fixed for AQ become extinct twice as often (i.e., λQ = 10%).
|
| *i) |
How much genetic diversity will there be within demes (a qualitative answer will be enough here). HINT 21AA
|
| **ii) |
How rapidly will allele AP increase in frequency? HINT 21BB
|
| ***iii) |
Suppose that AP is fixed throughout the population. Assume that allele AQ doubles extinction probability, as before, but also has an advantage within demes, because it increases relative fitness by s = 1%. What will happen? HINT 21CC
|
|
Problem 21.10
In the Prisoner’s Dilemma game, it always pays for any individual to “defect,” even though all would be better off if everyone cooperated (see Fig. 21.33). If individuals meet each other repeatedly, then more complex strategies become possible. In the tit-for-tat strategy, an individual cooperates on the first encounter, but if his/her opponents defect, he/she will defect when he/she meets that opponent again.
|
| *i) |
Using the payoff table of Figure 21.33, and assuming that individuals meet each other three times, find whether the tit-for-tat strategy can invade a population where all defect.
|
| *ii) |
Can a strategy of defection invade a population where all play tit-for-tat?
|
| **iii) |
What factors might help cooperation spread through a population where all individuals defect? HINT 21DD
|
